Jeannine Mosely: Curved Origami
Artist(s):
Title:
- Curved Origami
Exhibition:
Creation Year:
- 2008
Medium:
- paper
Category:
Artist Statement:
Folding along curves is very different from folding straight lines. The most important difference is that when you make a curved crease, the two parts of the paper that lie on opposite sides of the crease cannot be made to lie flat against each other. So while in conventional origami, facets of the paper become layered and hidden, in curved origami most of the paper remains exposed to view. This usually results in larger spans of paper that must be self supporting, so even the materials used are different: most origami paper is as thin as possible, while curved origami often requires very heavy paper or card stock.
I strive to create designs in which complexity arises from the interaction or repetition of simple shapes, so that the results are both immediately comprehensible and unexpected. I begin with experimentation: I put some creases in the paper, bend it and see what shapes interest me. Shall I repeat the shapes symmetrically or transition to a different shape? How will the regions interact with each other, and what can be done with the paper between two regions to allow a smooth transition, without stretching or crumpling the paper?
By modeling paper as an idealized mathematical surface and applying theorems from differential geometry, we can develop methods for analyzing and designing curved origami shapes. Paper can be represented as a “developable” surface, which means that no matter how you fold or bend it, at every point on the surface it is possible to embed a straight line in the surface passing through the point. Developable surfaces may be planes, generalized cylinders or cones, or tangent surfaces.
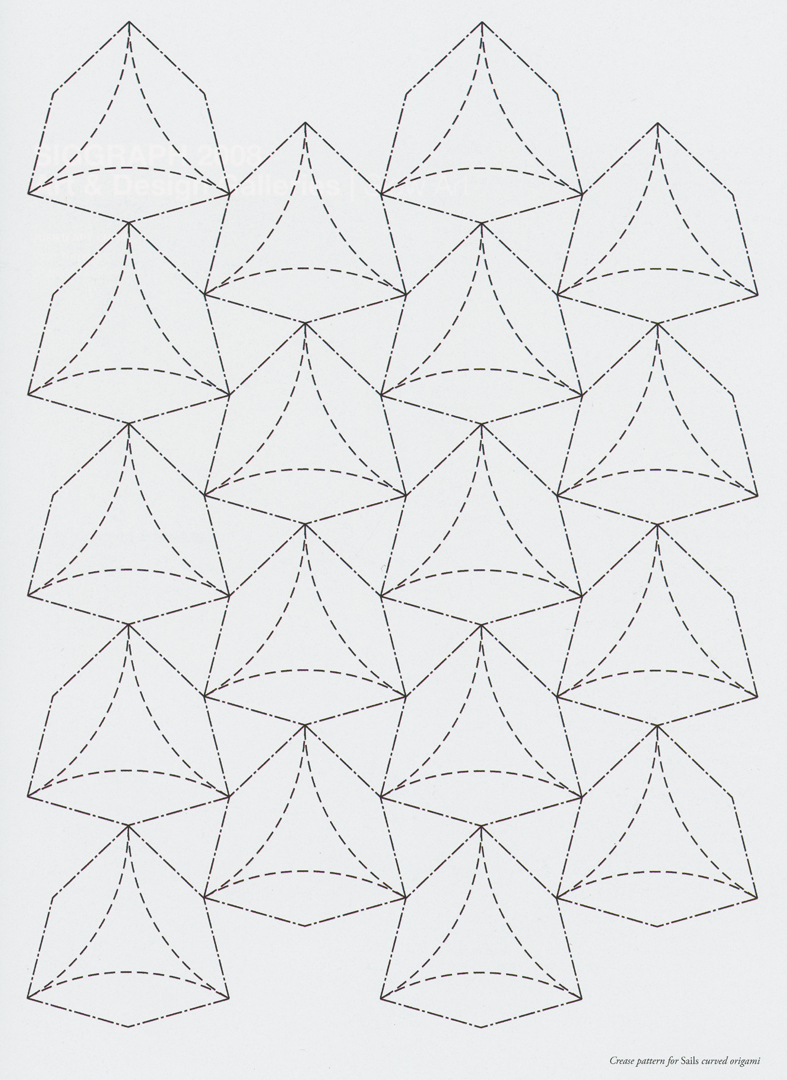
Intuition tells us that we cannot fold a sphere from a piece of paper, and yet the tops of the triangular pillows in Sails (see image and crease pattern) appear to be domed. Look closely, and you will see that they are in fact equilateral triangles with three tapering cylindrical sections attached. On the other side of the three curved creases are three cones that all come to a point below the center of the dome. These units are connected with a planar tiling of triangles. All the component surfaces are developable. Even for models where the curves are all circular arcs, like Sails, the length and position of those arcs cannot be calculated without computer assistance.
The constraints imposed to guarantee that the repeated units will tile the plane require us to numerically solve an equation containing an elliptic integral. Since the parameter solved for is not algebraic, we also need the computer to plot the resulting curves. I use Mathematica for these tasks.
Because of the malleability of paper, it is often possible to construct curved models that do not conform to theory, but I feel that such models incorporate a kind of lie that even the eye of the untutored viewer can detect, and that designs founded in mathematics have a greater appeal, as they reveal that truth visually.